

| Name | Vector Calculus | Tensor Analysis | Spacetime Algebra | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Lorenz gauge condition | \(\nabla\cdot\mathbf{A}+\frac{1}{c^2}\frac{\partial\varphi}{\partial t}=0\) | \(\partial_\mu A^\mu=0\) | \(\square\cdot A=0\) | ||||||
| Electromagnetic potential |
|
\(\partial_\mu\partial^\mu A^\nu=\mu_0J^\nu\) | \(\square^2 A=\mu_0J\) | ||||||
| Electromagnetic fields |
|
\(F^{\mu\nu}=2\partial^{[\mu}A^{\nu]}\) | \(F=\square\wedge A\) | ||||||
| Maxwell's equations |
|
|
\(\square F=\mu_0J\) | ||||||
| Continuity equation | \(\nabla\cdot\mathbf{J}+\frac{\partial\rho}{\partial t}=0\) | \(\partial_\mu J^\mu=0\) | \(\square\cdot J=0\) | ||||||
| Ohm's law | \(\mathbf{J}=\sigma(\mathbf{E}+\mathbf{v}\times\mathbf{B})\cosh\zeta\) | \(J^\mu=\sigma F^{\mu\nu}U_\nu\) | \(J=\sigma F\cdot U\) |
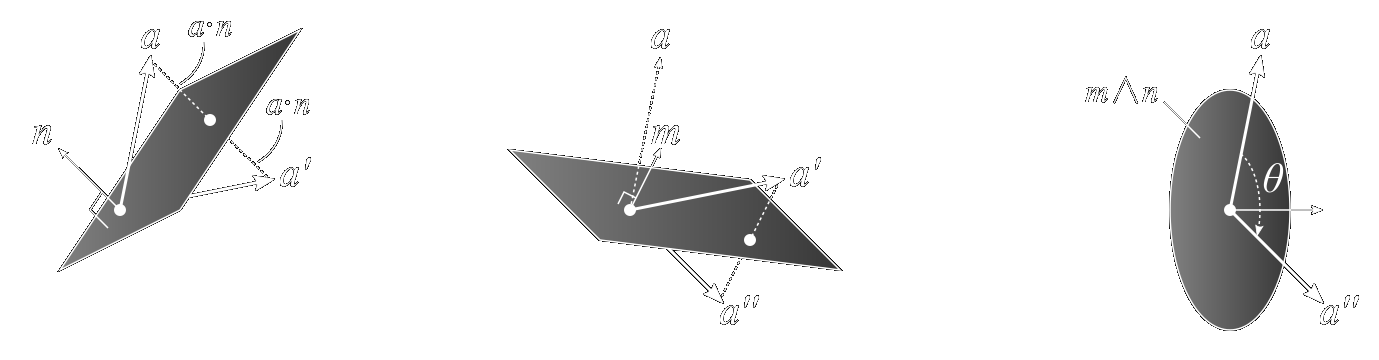
Vectors
Tensors
Spacetime Algebra
For more information about the relativistic form and application of these equations, the reader is referred to my book, Relativistic Field Theory for Microwave Engineers :
Now available at BookBaby and Amazon.com.