Next: Time Series Analysis of Unequally Spaced Data: Intercomparison Between Estimators of the Power Spectrum
Previous: Unified Survey of Fourier Synthesis Methodologies
Up: Algorithms
Table of Contents - Index - PS reprint
Astronomical Data Analysis Software and Systems VI
ASP Conference Series, Vol. 125, 1997
Editors: Gareth Hunt and H. E. Payne
V. L. Oknyanskij
Sternberg Astronomical Institute, Universitetskij Prospekt 13, Moscow, 119899, Russia
Abstract:
Time delay determinations in astrophysics are used most often to find
time delays between flux density variations in different spectral
bands and/or lines in AGNs, and different images of
gravitationally lensed QSOs. Here we consider a new algorithm for
a complex case, when the time delay is itself a linear function of
time and the intensity of echo response is exponential function of the
delay. We apply this method to the optical-to-radio delay in
the lensed double quasar Q0957+561.
Radio-optical variability correlation in Q0957+561 was first reported
by Oknyanskij & Beskin (1993, hereafter OB) on the basis of radio
observations made in the years 1979 to 1990. OB used an idea to take
into account the known gravitational lensing time delay to get
combined radio and optical light curves and then to use them for
determination of the possible radio-from-optical time delay. It was
found this way that radio variations (5MHz) followed optical ones
by about 6.4 years with high level of correlation (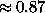 ).
Using new radio data (Haarsma et al. 1997), for the interval 1979-1994,
we find nearly the same value for the optical-to-radio delay as had
been found before. Additionally, we suspect that the time delay value
is linearly increasing at about 110 days per year while the portion
of reradiated flux in the radio response is decreasing.
).
Using new radio data (Haarsma et al. 1997), for the interval 1979-1994,
we find nearly the same value for the optical-to-radio delay as had
been found before. Additionally, we suspect that the time delay value
is linearly increasing at about 110 days per year while the portion
of reradiated flux in the radio response is decreasing.
We conclude that the variable radio source is ejected from the
central part of the QSO compact component.
Time delay determinations in astrophysics are used most often to
find time shifts between variations in different spectral bands and/or
lines in AGNs, as well as time delays between different
images of gravitationally lensed QSOs. In most cases, the task
is complicated by uneven spacing of data, so that standard
cross-correlation methods become useless. Two different methods
are most often used: CCF (Gaskell & Spark 1986) and DCF (Edelson &
Krolik 1988), which are based on line interpolation of
data sets or binning of correlation coefficients,
respectively. We have introduced
several simple improvements to CCF (Oknyanskij 1994) and this
modernized MCCF combines the best properties of CCF and DCF
methods. With MMCF we calculated regression
coefficients as functions of time shift. Here,
this calculation is
generalized for the more complex case where the time delay is a
linear function of time, and a portion of the flux density is
itself a power-law function of the delay. We apply this method to
the optical-to-radio time delay in the gravitationally lensed double quasar
Q0957+561.
The data sets used here were obtained to determine the
gravitational lensing time delay 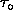 .
Our results are nearly identical
for values of
.
Our results are nearly identical
for values of 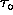 in the interval of 410-550 days.
In the
discussion below, we take
in the interval of 410-550 days.
In the
discussion below, we take 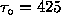 days.
days.
Our method includes several steps, which are briefly explained below:
- Combined light curves.
-
We take the radio (Haarsma et al. 1997) and optical (Vanderriest et
al. 1979; Schild & Thompson 1995) data sets for A and B images and
determine (using MCCF) the line regression coefficients k(
 )
and m(
)
and m( ). Then we transform
). Then we transform 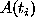 values into the B image
scale system for the known value of
values into the B image
scale system for the known value of 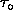 :
:
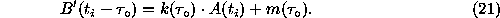
We combine these values 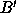 with the usual B ones, sorting
by time. The resulting optical light curve was
then smoothed by averaging in 200 day intervals with steps of 30
days. This accounts for the physical argument that radio
sources should be bigger than optical ones. The value of 200 days for
smoothing was taken as about optimal from the autocorrelation analysis
of light curves.
with the usual B ones, sorting
by time. The resulting optical light curve was
then smoothed by averaging in 200 day intervals with steps of 30
days. This accounts for the physical argument that radio
sources should be bigger than optical ones. The value of 200 days for
smoothing was taken as about optimal from the autocorrelation analysis
of light curves.
- Correction for change of time delay and radio flux.
-
Taking the optical-to-radio time delay
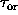 to be
a linear function of time, let V be the change of optical-to-radio
time delay
to be
a linear function of time, let V be the change of optical-to-radio
time delay 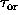 per year. We fix some moment of time as
per year. We fix some moment of time as
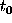 . It is attractive to choose
. It is attractive to choose 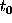 so that it falls near a strong
maximum in the optical light curve (here J.D. 2445350), which obviously
correlates with the high maximum in the radio light curve if take
so that it falls near a strong
maximum in the optical light curve (here J.D. 2445350), which obviously
correlates with the high maximum in the radio light curve if take
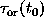 = 2370 days. So we can calculate the needed
correction:
= 2370 days. So we can calculate the needed
correction:
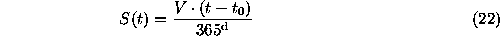
to be added to dates in the optical light curves:

Assuming that a portion of radio flux decreases as
a power-law function of time with exponent 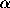 .
We should also correct the
optical flux for that fading before computing the cross-correlation
function:
.
We should also correct the
optical flux for that fading before computing the cross-correlation
function:
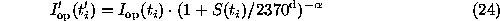
- Computing MCCFs.
-
We compute an array of MCCFs for combined radio and optical light
curves, varying V
and
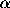 .
.
- Map cross-correlation as a function of V and
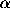 .
.
-
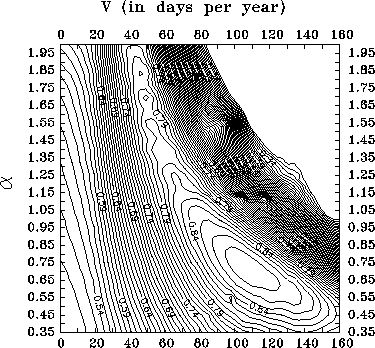
Figure: Two-dimensional cross-correlation function (see text).
Original PostScript figure (192kB).
For points 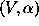 we map the MCCF values
(see Figure 1).
The best correlation
occurs for
we map the MCCF values
(see Figure 1).
The best correlation
occurs for 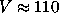 days/year, and
days/year, and  .
.
- Comparison of optical and radio light
curves.
-
We correct the optical combined light curve using (3) and (4) with the
parameters
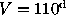 and
and  , shift ahead by
, shift ahead by
 , and then fit to the radio data
by analogy with (1). The corrected optical light curve is shown with
with the radio light curves in
Figure 2. Most features in both light curves coincide quite
well. So the investigation supports our assumption on the lengthening
of the optical-to-radio time delay. As a result we can give an
expression for the optical-to-radio time delay as a linear function of
time:
, and then fit to the radio data
by analogy with (1). The corrected optical light curve is shown with
with the radio light curves in
Figure 2. Most features in both light curves coincide quite
well. So the investigation supports our assumption on the lengthening
of the optical-to-radio time delay. As a result we can give an
expression for the optical-to-radio time delay as a linear function of
time:

We have calculated the time delay between radio and optical flux
variations using a new method. In addition, we have investigated the
possibilities that (1) there is a change of the time delay that is a
linear function of time, and (2) the radio response has power-law
dependence on the time delay value.
Finally, let us stress some additional consequences from our results:
- For some objects, optical-to-radio time delays
were probably not found because they were too long
compared to the duration of monitoring program.
- Optical-radio correlations may not have been recognized in some
objects since the time delays as well as response functions probably
were variable. This possibility has never been entertained before.
- The variable radio flux in Q0957+561 may originate
in a very compact jet component
moving away from the optical source.
Only after another jet component appears (whose
time delay value will of course be different) will the QSO again show
some optical-radio correlation.
- If several compact jet components exist simultaneously in a QSO
then we may have no chance to find any radio-optical correlation. Only
if these jet components move toward the observer closely to the line
of sight (as it is probable for Blazars), will radio-optical
correlation have a chance to be found, since the time delays
for all these variable radio components would be very close to zero.
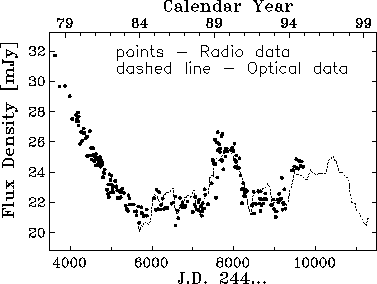
(The optical light curve is corrected as described in the text.)
Original PostScript figure
(168kB).
Acknowledgments:
In conclusion, we thank Debborah Haarsma for sending us the preprint
with the new radio data for Q0957+561 before publication.
References:
Edelson, R. A., & Krolik, J. H. 1988, ApJ, 333, 646
Gaskell, C. M., & Spark, L. S. 1986, ApJ, 305, 175
Haarsma, D. B., Hewitt, J. N., Lehar, J., & Burke, B. F. 1997, ApJ, 479, 102
Oknyanskij, V. L., & Beskin, G. M. 1993, in Gravitational
Lenses in the Universe: Proceedings of the 31st Liege International
Astrophysical Colloquium, eds. J. Surdej et al. (Liege, Belgium:
Universite de Liege, Institut d'Astrophysique), 65
Oknyanskij, V. L. 1994, Ap&SS, 222, 157
Schild, R. E., & Thomson, D. J. 1995, AJ, 109, 1970
Vanderriest, C., et al. 1989, A&A, 215, 1
© Copyright 1997 Astronomical Society of the Pacific,
390 Ashton Avenue, San Francisco, California 94112, USA
Next: Time Series Analysis of Unequally Spaced Data: Intercomparison Between Estimators of the Power Spectrum
Previous: Unified Survey of Fourier Synthesis Methodologies
Up: Algorithms
Table of Contents - Index - PS reprint
payne@stsci.edu
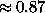 ).
Using new radio data (Haarsma et al. 1997), for the interval 1979-1994,
we find nearly the same value for the optical-to-radio delay as had
been found before. Additionally, we suspect that the time delay value
is linearly increasing at about 110 days per year while the portion
of reradiated flux in the radio response is decreasing.
).
Using new radio data (Haarsma et al. 1997), for the interval 1979-1994,
we find nearly the same value for the optical-to-radio delay as had
been found before. Additionally, we suspect that the time delay value
is linearly increasing at about 110 days per year while the portion
of reradiated flux in the radio response is decreasing.
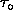 .
Our results are nearly identical
for values of
.
Our results are nearly identical
for values of 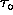 in the interval of 410-550 days.
In the
discussion below, we take
in the interval of 410-550 days.
In the
discussion below, we take 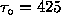 days.
days.
 )
and m(
)
and m( ). Then we transform
). Then we transform 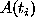 values into the B image
scale system for the known value of
values into the B image
scale system for the known value of 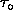 :
:
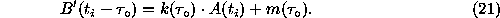
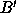 with the usual B ones, sorting
by time. The resulting optical light curve was
then smoothed by averaging in 200 day intervals with steps of 30
days. This accounts for the physical argument that radio
sources should be bigger than optical ones. The value of 200 days for
smoothing was taken as about optimal from the autocorrelation analysis
of light curves.
with the usual B ones, sorting
by time. The resulting optical light curve was
then smoothed by averaging in 200 day intervals with steps of 30
days. This accounts for the physical argument that radio
sources should be bigger than optical ones. The value of 200 days for
smoothing was taken as about optimal from the autocorrelation analysis
of light curves.
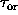 to be
a linear function of time, let V be the change of optical-to-radio
time delay
to be
a linear function of time, let V be the change of optical-to-radio
time delay 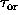 per year. We fix some moment of time as
per year. We fix some moment of time as
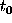 . It is attractive to choose
. It is attractive to choose 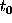 so that it falls near a strong
maximum in the optical light curve (here J.D. 2445350), which obviously
correlates with the high maximum in the radio light curve if take
so that it falls near a strong
maximum in the optical light curve (here J.D. 2445350), which obviously
correlates with the high maximum in the radio light curve if take
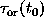 = 2370 days. So we can calculate the needed
correction:
= 2370 days. So we can calculate the needed
correction:
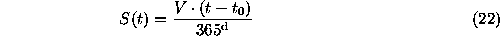

 .
We should also correct the
optical flux for that fading before computing the cross-correlation
function:
.
We should also correct the
optical flux for that fading before computing the cross-correlation
function:
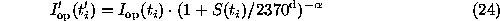
 .
.
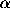 .
.

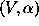 we map the MCCF values
(see Figure
we map the MCCF values
(see Figure 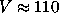 days/year, and
days/year, and  .
.
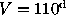 and
and  , shift ahead by
, shift ahead by
 , and then fit to the radio data
by analogy with (1). The corrected optical light curve is shown with
with the radio light curves in
Figure 2. Most features in both light curves coincide quite
well. So the investigation supports our assumption on the lengthening
of the optical-to-radio time delay. As a result we can give an
expression for the optical-to-radio time delay as a linear function of
time:
, and then fit to the radio data
by analogy with (1). The corrected optical light curve is shown with
with the radio light curves in
Figure 2. Most features in both light curves coincide quite
well. So the investigation supports our assumption on the lengthening
of the optical-to-radio time delay. As a result we can give an
expression for the optical-to-radio time delay as a linear function of
time:

