The correlation shown in Figure 3, the slow (zero) spreading rate of the jet, and the constant separation of the "rails" are consistent with a jet whose structure is approximately self-similar everywhere between J1 and J4. We therefore modeled the averaged transverse I and P profiles, rather than individual profiles, to improve our signal-to-noise.
We use 
coordinates in a cylindrical jet axisymmetric
about the z axis and inclined by angle i to the plane
of the sky. The jet is populated by relativistic electrons with a power-law
energy distribution, a specified emissivity distribution,
and a specified magnetic field geometry, including both organized and
random components. The transverse I, Q and U profiles are
computed by integrating 1301 equal cells along each of 131
equally-spaced lines of sight through the jet, and are then
convolved to the resolution of our data. We
matched the index of the power-law electron energy distribution
to the flattest spectral index associated with the jets
(
); our results are insensitive to this choice.
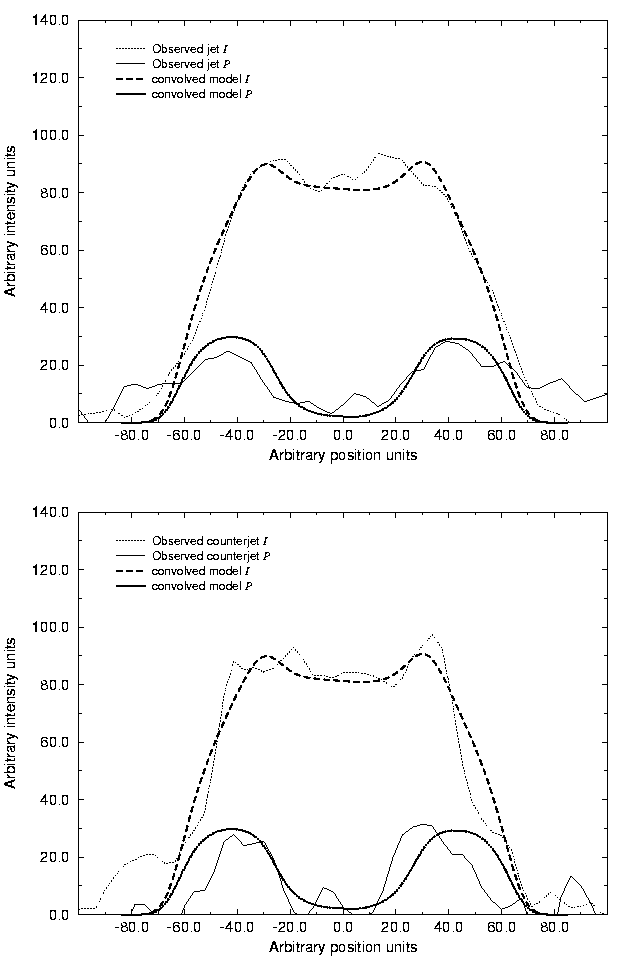
Figure 4: Predicted transverse I and P profiles of the model with zero emissivity in the spine, whose boundary is here at r=26 (0.43 that of the jet), compared with the observed profiles. The model profiles have been convolved to the same  FWHM resolution as the observations of the jet (upper panel)
and counterjet (lower panel).
|
Figure 4 shows the average transverse I and P profiles that we infer by assuming that the lobe magnetic field is perpendicular to both jets everywhere. These profiles are fitted well by a model (also shown) in which
of the radius of the jet),
, 
(toroidal) and 
(axial) in the layer
are uniformly distributed zero-mean random variables normalized so that
.
The value of
in this model is a compromise
between the optimal fits for the jet and the counterjet.
For the jet, the ranges of parameters that fit the observed I
and P profiles within their 2
errors everywhere,
holding the other parameters fixed, are
,
,
. The best-fitting values of
in the counterjet are 
% smaller.
Such models with no emission from the jet "spine''
at 
predict the observed I profiles to within
their errors, but in detail the model profiles
are too edge-brightened. Better fits are obtained if the spine
also radiates, but with an emissivity less than half that of the
outer layer, and if the spine field has 
.
The constraints imposed by fitting the I and P profiles
simultaneously are strong, so it is unlikely that
any fundamentally dissimilar models of magnetic field organization and
relative emissivity will fit our data so well. A smaller ratio of
to can however be traded against orienting the
jet away from the plane of the sky to some extent: models with
as the only field component in the radiating layer can
reproduce the observed flat-topped I profiles, but
they over-predict the degree of linear polarization at the rail centers,
typically by a factor of two.
We also computed the emission from several well-ordered field structures previously proposed for large-scale jets, including helical fields and transverse self-similar "flux ropes'' (Chan & Henriksen 1980). We found none that simultaneously produced flat-topped I profiles and symmetric P profiles with ~ 20 to 30% polarization (Swain 1996).